P6: Adjoint geodynamic model enabled by high-performance computing

Project leader
Hamish Brown
Supervisors
Prof. Hans-Peter Bunge, Prof. Dieter Kranzlmüller
Advisors (int.)
Dr. Bernhard Schuberth (LMU)
Advisors (ext.)
Asst. Prof. Lorenzo Colli (Univ. Houston)
Description
The geodynamic model serves as one of the key elements in PC IV: the coupled process model. We use the novel adjoint method to determine a trajectory of past mantle flow that is in accordance with present day’s observations from seismic tomography.
Based on fundamental physical principles, we can identify flow and flow changes with a global stress field and thus, with uplift rates over geological time scales. The generated uplift rates from this project form the basis of the coupled physical process model and especially serve as an input / coupling to the landscape model (P7). Observations from geodesy (P1, P2) and geology (P3, P4) will be used in a revalidation process for the assumed physical parameters of the model. Especially, we will gain insights to the Earth’s viscosity profile, which has now been under debate for many decades.
Motivation
Global models of past mantle convection have become a common tool in geodynamics. However, the significant uncertainties of the input model parameters necessitates new observational datasets with which to test the resulting models. To this end, I use inferences of past and present dynamic topography to assess mantle circulation models. This allows me to explore different choices for the mantle viscosity structure and reduce the vast uncertainties in this parameter through comparison with surface observations.
Main objectives
- Upgrade of (existing) geodynamic Earth models to the exascale
- Assimilation of seismic tomography data via the adjoint method
- Determination of model sensitivities to input parameters
- State of the art visualization of model output and observational data sets (in connection with P10)
RTG coupling
Input:
- Present-day mantle heterogeneity (‘terminal condition’) derived from seismic tomography (P5).
- Backward uncertainty estimates from P9.
- Plate motion history as a velocity boundary condition (external).
- Mineralogy (conversion between material parameters, i.e. seismic velocities, density, temperature) (external)
Output:
- Trajectory of Earth mantle’s temperature structure from past to present.
- Trajectory of Earth mantle’s density structure from past to present.
- Trajectory of Earth mantle’s stress field from past to present (P8).
- Trajectory of Earth’s gravity field (mantle contribution) from past to present (to P2, P7, P8).
- Trajectory of Earth’s dynamic topography induced by mantle flow from past to present (to P3, P5, P6, P7, P8).
- Sensitivity of model parameters and uncertainty quantification (in connection with P9).
- In particular, all generated data will be provided for visualization purposes (P10).
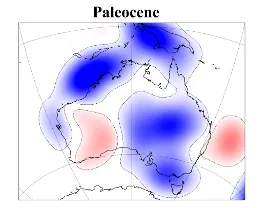
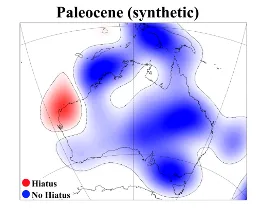
Preliminary results
Hiatus maps are an important new inference of past dynamic topography. I have been able to generate synthetic hiatus maps by converting model outputs into synthetic geological data. Comparison of several models to the observed hiatus maps has shown that a thin, weak asthenosphere is an essential aspect of the radial mantle viscosity structure.
Presentations
“Synthetic Hiatus Maps as a Tool for Constraining Global Mantle Circulation Models” - Talks given at UK-SEDI 2024, Leeds, and EGU 2024, Vienna